Citation: Toole K, Hutton B, McLusky J, “Defining High-Viscosity, Large-Volume Drug Delivery Systems”. ONdrugDelivery, Issue 166 (Oct 2024), pp 47–50.
Kyle Toole, Blair Hutton and James McLusky discuss the value that mathematical modelling can provide in the early stages of injectable drug delivery device development, especially when coupled with appropriate empirical testing in a laboratory setting.
Recent advancements in biologics have led to breakthrough therapies for a variety of indications. However, these formulations often require higher viscosities and larger volumes for administration, which presents significant challenges for the development of new drug delivery devices for subcutaneous injection. Simultaneously, these devices must meet the broader needs of both patients and healthcare providers.
Meeting these needs requires the consideration of core factors such as patient tolerability, the formation and dispersion of the subcutaneous depot, device usability, choice of primary container and the technical capabilities of the delivery device itself. One crucial performance requirement that influences the ability of any device to address these factors is the injection duration and the forces required to achieve it.
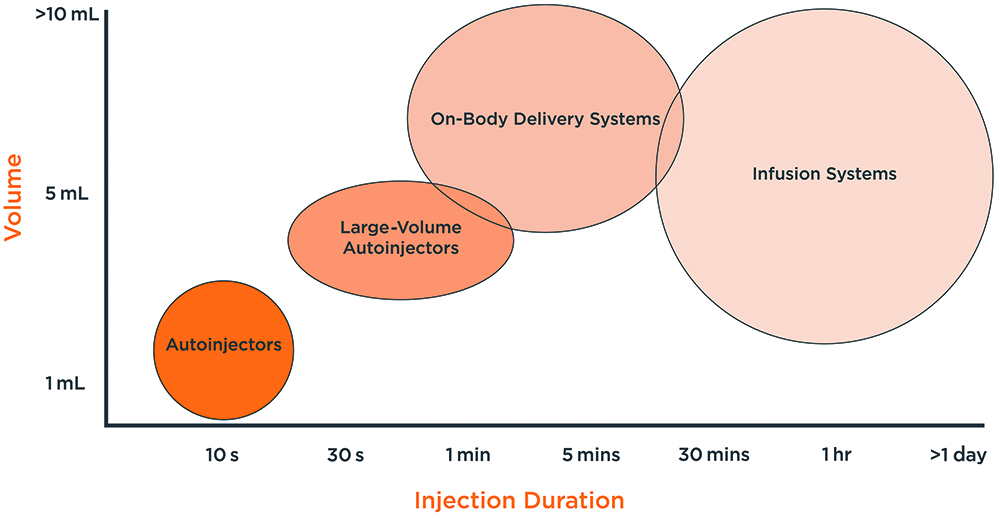
Figure 1: The drug delivery device landscape.
As shown in Figure 1, there are multiple delivery systems available, or emerging, within the drug delivery landscape. Using mathematical modelling and explorative laboratory testing early in the development process, an engineering team can quickly understand the capabilities and limitations of an established device or the need for a new concept in relation to a new drug formulation. This insight can enable the efficient evaluation of a concept, helping to define the delivery strategy and design of next-generation drug delivery systems with informed decisions. Mathematical modelling and explorative laboratory testing can allow engineers to:
- Define the most suitable device format for a concept, along with the associated primary container and needle combination
- Optimise the often-conflicting design variables of a concept
- Determine if a concept can still perform at its operating limits.
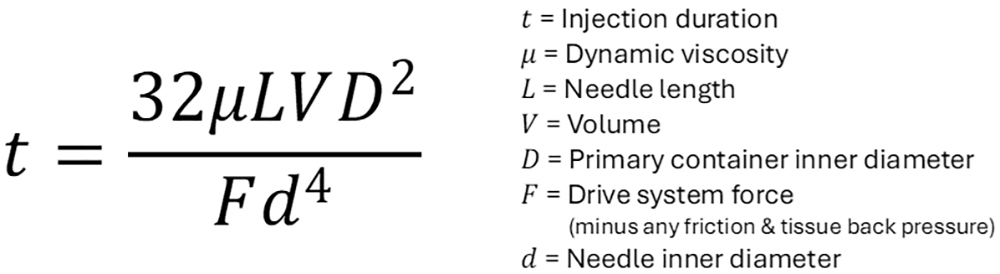
Figure 2: Modified Hagen-Poiseuille equation and terms.
MATHEMATICAL MODELLING
Using a modified Hagen-Poiseuille equation (Figure 2), it is possible to calculate the nominal injection duration for a given configuration. This equation effectively models injection duration for high-viscosity Newtonian formulations,producing reasonably accurate results. Before moving on, it should be noted that non-Newtonian fluids, such as formulations that exhibit shear thinning behaviour, require alternative models.
The parameters of the modified Hagen-Poiseuille equation are:
- Viscosity and volume, including environmental factors
- Needle selection
- Primary container
- Plunger stopper friction
- Tissue back pressure
- Drive system force.
“Many biologics are sensitive to temperature changes, so using ‘temperature versus viscosity’ data in the model helps predict how injection duration might vary in actual use.”
Viscosity and Volume
Known or theoretical viscosity and volume data are added into the model to assess how they impact injection duration across different delivery device concepts. Environmental factors should also be considered here – whilst room temperature is often controlled in clinical settings, patients administering at home will encounter temperature ranges that can significantly affect viscosity, even after accounting for warm-up time post-refrigeration. Many biologics are sensitive to temperature changes, so using “temperature versus viscosity” data in the model helps predict how injection duration might vary in actual use. These insights can be used to refine the device design and inform any mitigating steps that may be added to the instructions for use (IFU).
Needle Selection
Choosing the right needle has long been understood as critical for both patient comfort and effective drug delivery. The needle’s gauge, wall thickness and length significantly influence fluid flow. Smaller diameter needles reduce patient discomfort but can restrict fluid flow and may increase the risk of occlusion. Conversely, larger diameter needles improve fluid flow but increase patient discomfort.
The Hagen-Poiseuille equation clearly shows that flow rate is inversely proportional to the fourth power of the needle’s inner radius, emphasising how needle diameter affects injection duration. Balancing these factors increasingly points towards the use of thin-walled or ultra-thin-walled needles. What may look like a small increase in bore diameter can have a huge impact on injection duration (Figure 3).
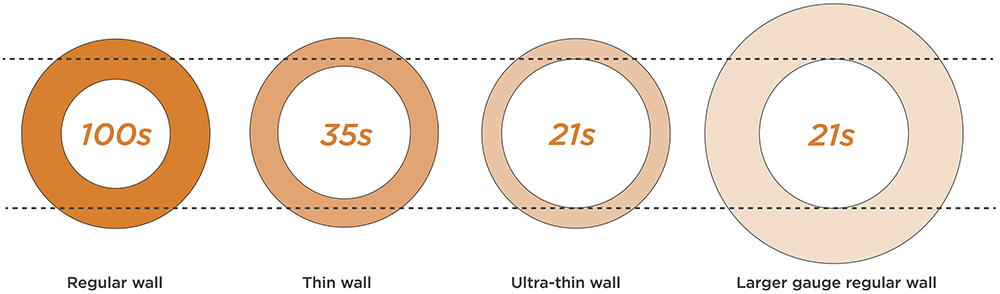
Figure 3: Illustrative impact on injection duration (in seconds) as needle bore diameter increases.
Primary Container
The inner diameter of the primary container is crucial because it influences the force required to expel the formulation. Larger container diameters may require more force to achieve a target injection duration as the applied pressure is equal to the force divided by the area, therefore increasing the burden on the delivery device. Additionally, the primary container’s aspect ratio – whether it has a large diameter with a short length or a small diameter with a long length – affects the device’s overall format and usability. Whilst numerous other factors can influence the selection of a primary container, an early evaluation of how it integrates into a device and its associated impacts on performance and usability can assist in making efficient decisions.
Plunger Stopper Friction
The glide force of the plunger stopper moving through the primary container is influenced by the stopper material and design, barrel material, surface treatment and level of lubrication. Known or expected values can be incorporated into calculations to account for the additional forces that the device must overcome to achieve the target injection duration.
Tissue Back Pressure
A factor that is often overlooked during the characterisation of a concept is the tissue back pressure. Typically, combination products are tested by “injecting” into free air. However, injecting into a patient’s subcutaneous tissue introduces back pressure, which can increase both the nominal injection duration and its variability, especially with high-viscosity, large-volume formulations.
Factors influencing tissue back pressure include tissue characteristics at the injection site, formulation composition, viscosity, volume and flow rate. Analysing data from literature and specific studies can enable the incorporation of tissue back pressure values into the equation, improving the understanding of its impact on injection duration.
Drive System Force
The Hagen-Poiseuille equation can also be rearranged to focus on the required force to achieve a given injection duration. This can enable a design team to evaluate concepts for the device form factor and determine suitable drive system technology options quickly. This can ensure that the selected concept will meet performance requirements and remain aligned to the overall programme strategy.
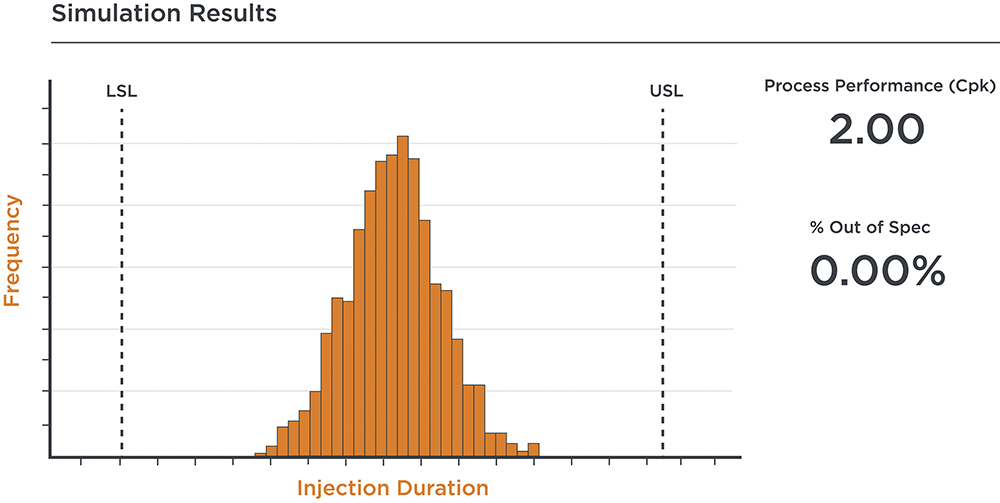
Figure 4: Stylised output from a Monte Carlo simulation for injection duration.
MONTE CARLO SIMULATION
Integrating the Hagen-Poiseuille equation within a probabilistic mathematical model can expand an engineering team’s understanding of a concept’s capabilities and limitations by accounting for potential variations and production tolerances. A Monte Carlo simulation is a powerful tool to help in this evaluation.
Where the Hagen-Poiseuille equation offers predictions under nominal conditions or simple edge cases, Monte Carlo simulations can extend this by modelling a broad range of scenarios. This method generates a predictive distribution or bell curve that reveals potential variations in injection duration and identifies the performance limits (Figure 4). Analysis using this approach can illustrate how different device configurations may perform under diverse conditions, thereby identifying potential issues. This simulation step ensures a robust concept throughout the development process with a view to high-volume manufacture.
“Whilst mathematical modelling is invaluable for exploring concepts and understanding any limitations, empirical data from laboratory testing always provides the most reliable insights.”
EXPLORATIVE LABORATORY TESTING
Whilst mathematical modelling is invaluable for exploring concepts and understanding any limitations, empirical data from laboratory testing always provides the most reliable insights. Early in the development process, the fluid path can be simulated using off-the-shelf components to compare against the theoretical data.
Equipment such as a universal test machine or syringe pump can simulate a drive system under nominal, best-case and worst-case conditions (Figure 5), whereas in-line pressure monitoring systems can record pressure data. This set-up, used in a suite of tests or design of experiments studies, can offer valuable insights into the forces and pressures required to achieve target injection durations.

Figure 5: Equipment such as a universal test machine or syringe pump can simulate a drive system.
If the drug product formulation is not yet available or if a range of viscosities need to be explored, viscosity mimics can be prepared. These mimics, verified with a viscometer, can be filled into the primary container or fluid path for testing. This approach not only fosters confidence in the mathematical models but can also help to identify any discrepancies or unexpected challenges early on in the design process. Following this initial exploratory testing, physical prototypes of the delivery device can be designed and integrated into development rigs, paving the way for the subsequent design and development stages.
TACKLING THE CHALLENGE OF HIGH-VISCOSITY, LARGE-VOLUME DRUG DELIVERY DEVICES
The development of delivery devices for the subcutaneous administration of high viscosity, large-volume biologics benefits from an integrated approach that combines mathematical modelling with empirical testing. Using tools such as the Hagen- Poiseuille equation and Monte Carlo simulations early in the development process can help to guide the selection of the optimal device format, balance design variables and assess performance limits.
Complementing these theoretical models with explorative laboratory testing can build confidence in the predictions and evaluate real-world performance. This combined approach significantly strengthens the likelihood that a drug delivery concept will meet the stringent performance requirements as it progresses through the development process toward high-volume production.
Finally, this approach is not only useful for informing new delivery system developments – which can be prohibitively expensive – the early engagement with device designers in any pharmaceutical formulation process can enable options that may or may not be suitable for existing platform devices and categories to be quickly reviewed. This can potentially expose the need to explore lower concentrations at larger volumes or higher concentrations at smaller volumes in a timely manner. Whatever the overall strategy, this considered approach can optimise therapeutic efficacy, improve the patient experience and significantly reduce commercialisation costs.

